

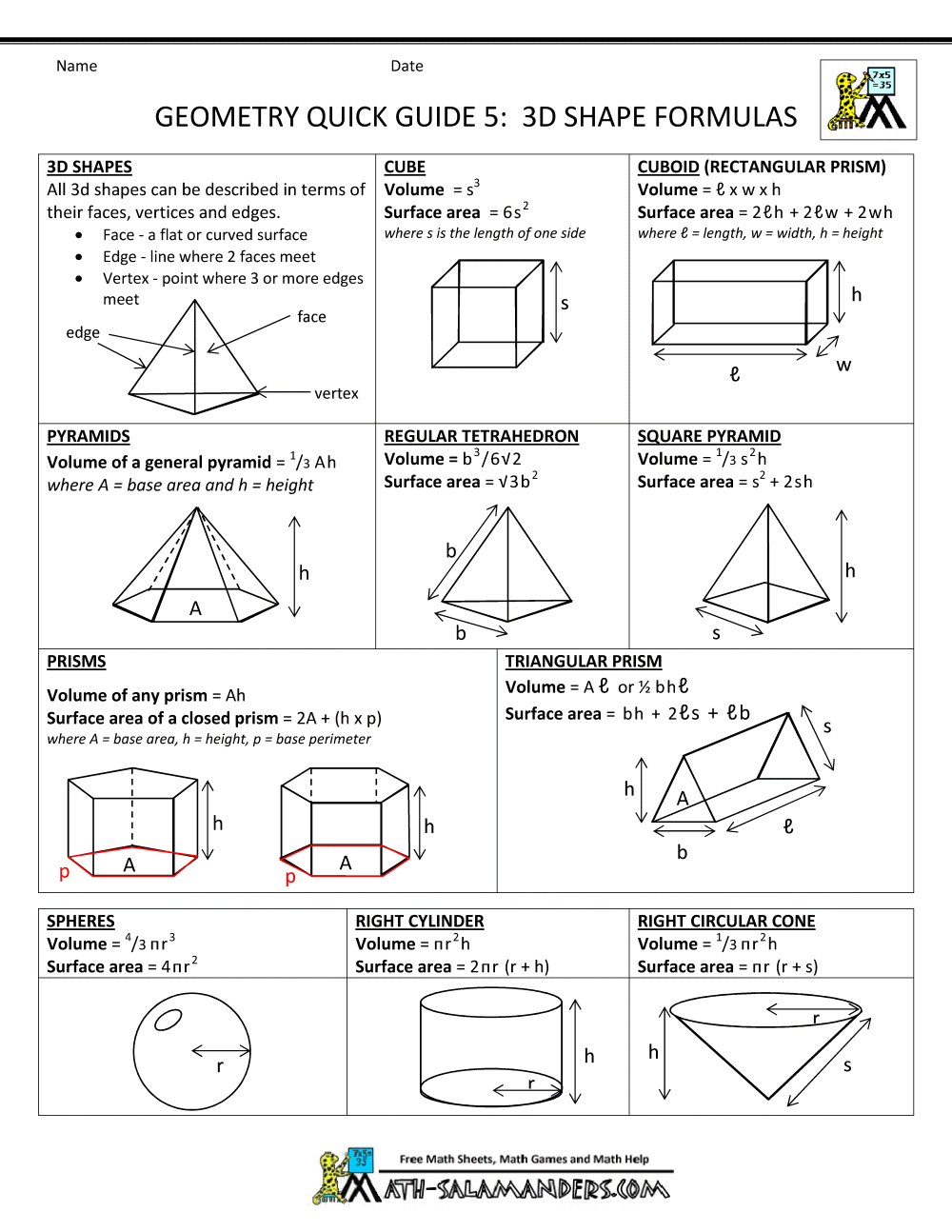
This theorem implies right angled triangles. In every triangle, longer side is opposite the larger angleĪnd smaller side opposite to the smaller angle. Triangles, the base should be extended in order to draw altitude.Īltitude is also called as height of the triangle.īh. In some cases like in the case of an Obtuse angled Triangle bisect the angle and the base too.įor a right angled triangle with right angle any side on theīase, altitude is measured as the length of the perpendicular. The altitude of an equilateral triangle and an isosceles The sum of all the 3 angles of a triangle => A + B + C = 180Īn altitude of a triangle is the line joining the base One of theĪngles = 90 degrees and the longest side is called ‘hypotenuse’ opposite to the right angle. If a triangle has one right angle, then it is said to be a Right Angled Triangle.

Transversal, there are three major angle relations When parallel lines are intersected by a.The shortest distance from a point to a line is.Two parallel lines have an equal slope.
Perpendicular lines meet at right angles.Two angles are complimentary if their angle sum.Two angles are supplementary if their angle sum is 180 degrees.When two lines meet at a point, pairs of opposite angles are equal.The angle from 180 to 360 is called reflex angle.An obtuse angle limits from 90 to 180 degrees.Acute angle limits from 0 to 90 degrees.As a line has no ends, two intersecting lines subtend 4 angles in total. Two lines intersecting at a point subtends an ANGLE on one side and that point is called VERTEX.There are 3 major pairs of lines they are Intersecting lines, perpendicular lines and.
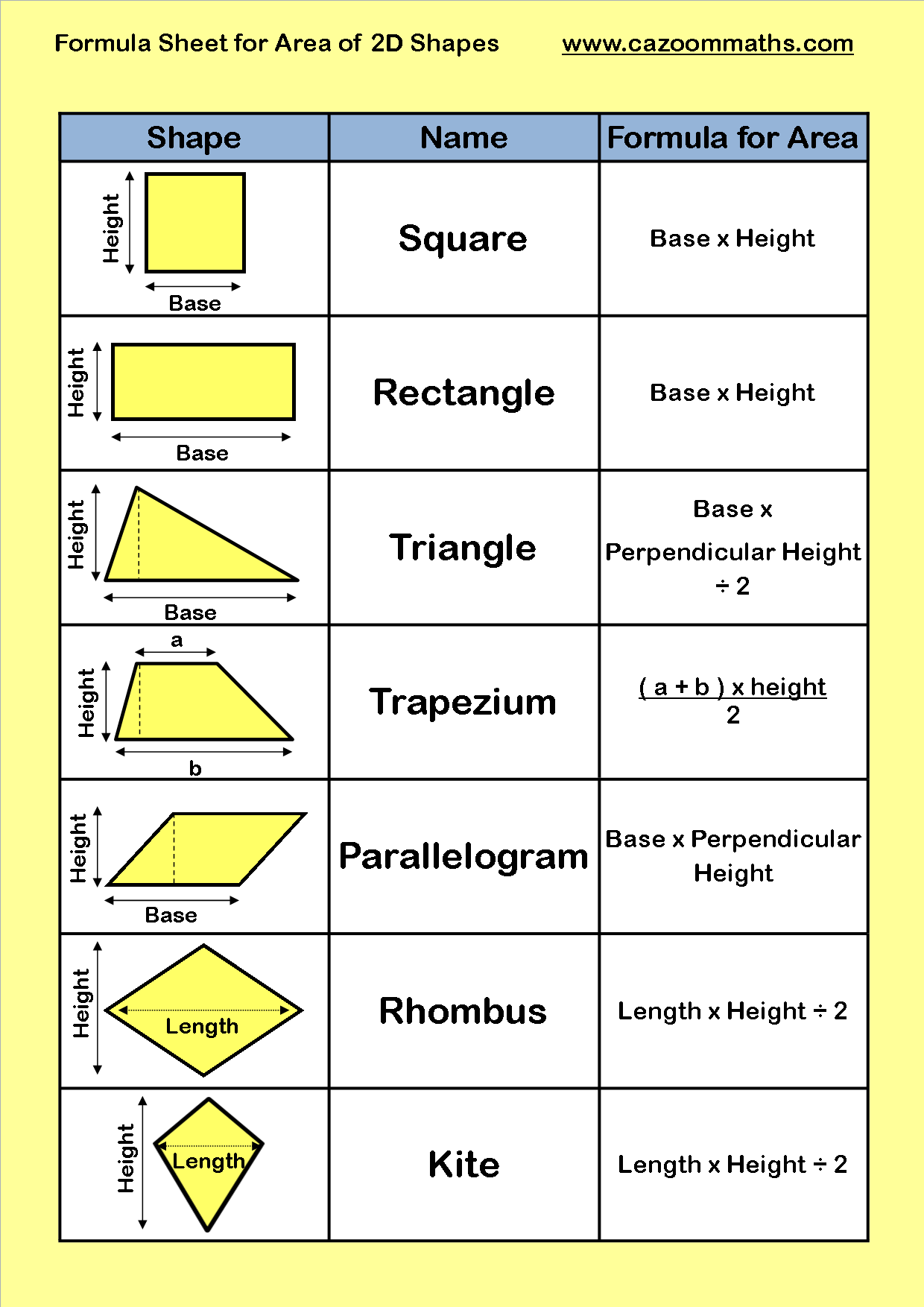
Segments are used in geometrical constructions (in diagrams as well). A ray originates from a point and extends in.A straight line with now end points is called a.(this regards practice) and you are ready for geometry now.Īs said definitions first. Whatever the calculation it is, if it is geometry, then To become successful in geometry, we have some set Because, most of the students have a misconception that GRE quant geometryĬan be solved just by eyeballing. GRE Geometry Concepts and FormulasĪll know is easy to understand but crisis generally arises when you solve a This is a pocket notes for reference at a glance. Today we feel pleasure to give you ‘the complete guide to geometry in GRE quant’ in brief. GRE quant comprises both arithmetic and geometry. Problems in which the student have to enter their own answers.įact is that all know that GRE quantitative reasoning In addition to this, the test does have numeric entry In general, 2 types of questions appear in GRE quant. Math in GRE has 2 sections with each section containing 20 questions each and the total time given is only 35 minutes. A little focus on this chapter will boost up your score exponentially. Every math student knows that Geometry is a scoring chapter. Geometry in GRE Quant is quite crucial where time is always a crisis.


 0 kommentar(er)
0 kommentar(er)
